II- Review of the Nature Principles (cont.)

g) Life

Life is everywhere.

Live can be found in all environments, continental, subaerial, aerial, desertic, fluvial, shallow marine, depth marine, even in hadal depths.

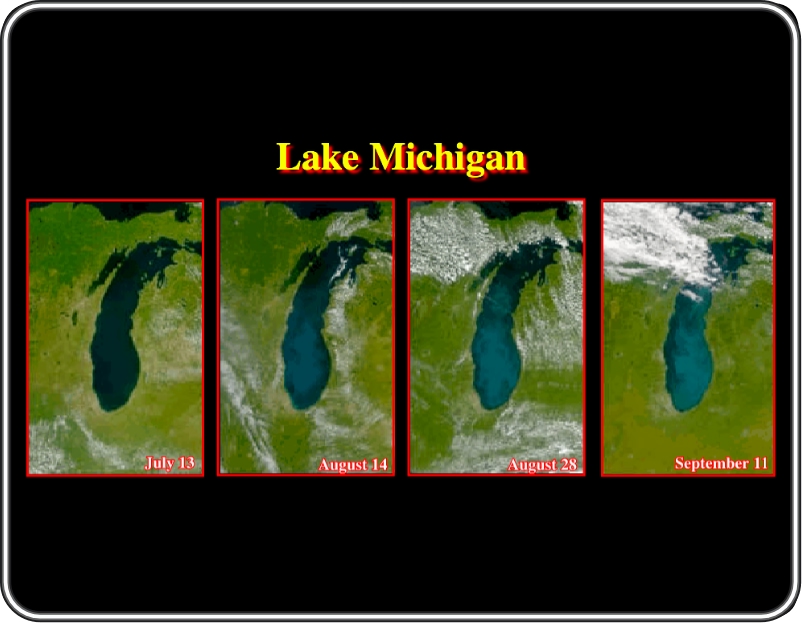
The bright color that appears in late summer in Lake Michigan is caused by the calcium carbonate in the water and not by life. Lake Michigan always has a lot a calcium carbonate because the floor of the lake is limestone. During most of the year the calcium carbonate remains dissolved in the cold water, but at the end of summer the lake warms up, lowering the solubility of calcium.

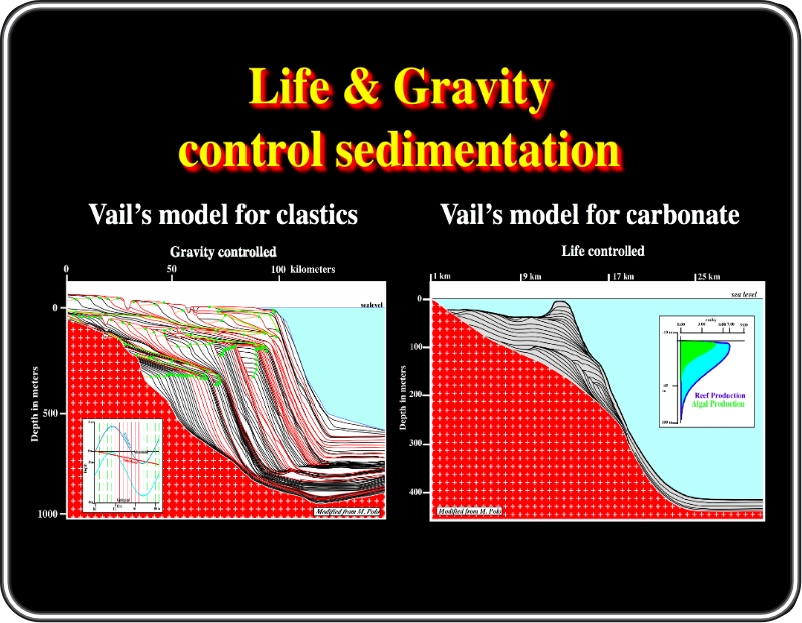
The carbonate depositional model of Exxon is illustrated on right was built using Marco Polo software. The parameters Eustasy and Subsidence are exactly the same that those used in the sand-shale model, also, the relative sea level curve is the same in both models. The only difference is the sedimentary input. In the sand-shale model, the sediments are transported from the continent toward the sea and the terrigeneous input is conventionally taken as constant, in the carbonate model, the algal and reef production are maximal (5-7 cm/ky) near surface at 2-3 meters water depth.

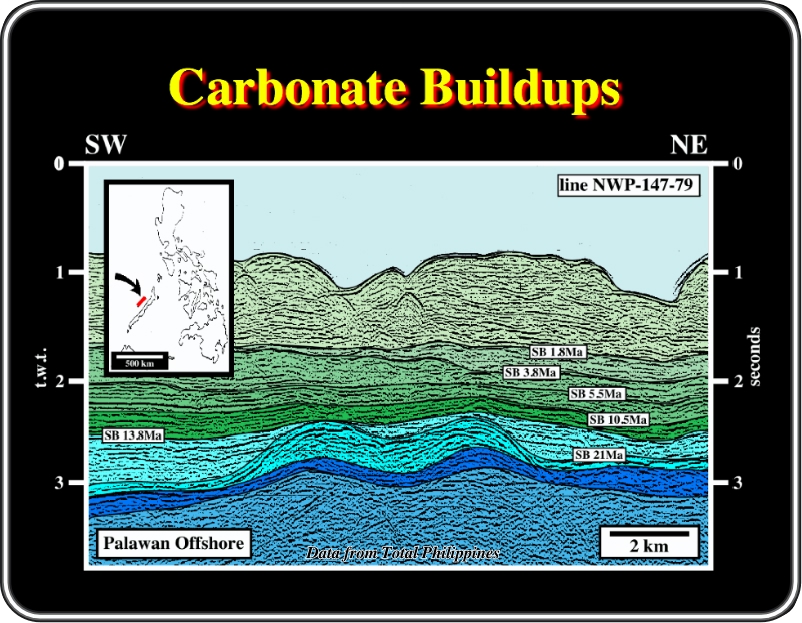
Carbonate buildups, as those illustrated on this line from off as other organic rocks obey to the three basic principles controlling life that we will review next.

Life is controlled by three major laws:

a) Fermat-Maupertius’s law
                   or Principle of minimum action.
b) Snell’s law
                   or Principle of minimum time.
c) Fibonacci’s law
                  or Principle of maximum room.

a)Fermat-Maupertius’s law

“Entia non sun multiplicanda praeter necessitatem”

Entities should not be multiplied unnecessarily.

Ockham, W., (1290-1349)

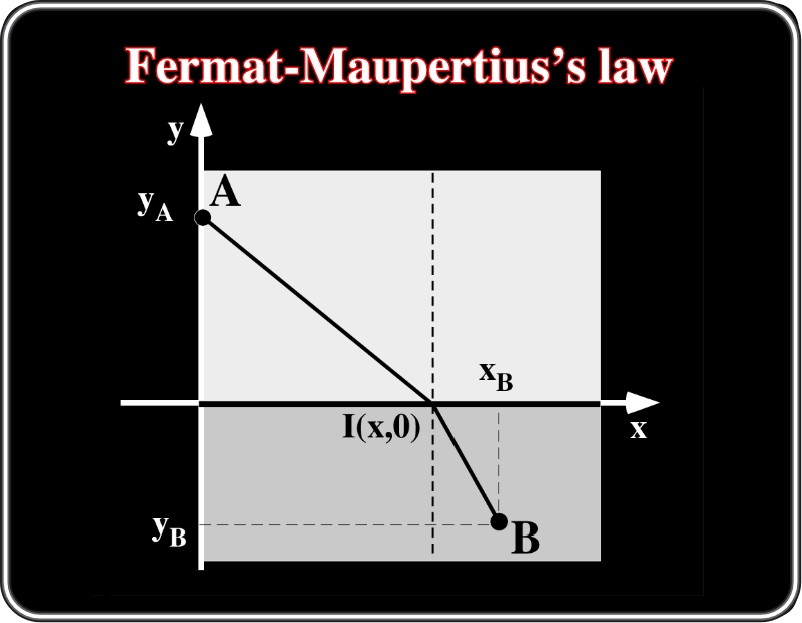
Pierre de Fermat (1601-1665) postulated the luminous rays followed a general principle according which the path borrowed by light to go from a given point to another was that for which the time of course was a mini-mum. En 1744, P. de Maupertius put forward that physical events followed a basic principle: “Nature follows always, among all possibilities, the more efficient one”. In the case of movement, for instance, such efficiency is corresponds to the minimum velocity for the minimum of traversed path. (Principle of minimum action).

b) Smell’s law

During refraction of light, the ratio of the sine's of the angles of incidence (i1) and refraction (i2) is a constant equal to the refractive index of the medium.

The reflection case illustrates a point about Format's principle: the minimum time may actually be a local rather than a global minimum. Trajectories allowed by Format's principle don't strictly have to be minimum time trajectories. They can also be maximum time trajectories.

c) Fibonacci’s law

In a Fiobonacci sequence each term (after the first two) is the sum of the preceding two: 1, 1, 2, 3, 5, 8, 13, 21, 34, 55...

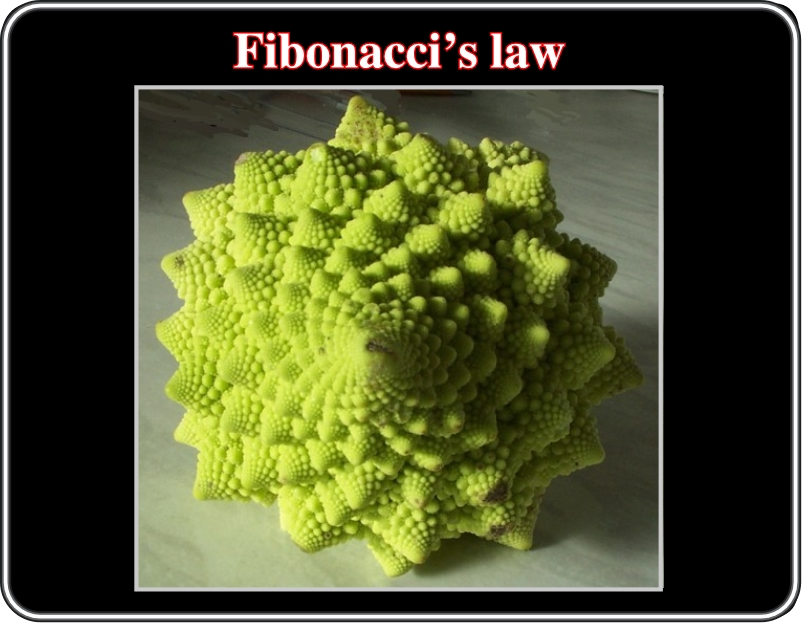
Each floret is peaked and is an identical but smaller version of the whole thing and this makes the spirals easy to see.

This simple pattern can determine the shape of a seashell, pine-cones or the sprouting of leaves from the stem of any plant. This connection to the organic world is reflected in the surface pattern, which is achieved through the process of smoke firing.

Fiona sequence has many other interesting mathematical properties. For example, the ratio od successive terms (larger to smaller; 1/1, 2/1, 3/2, 5/3, 8/5, 13/8....) approaches the number 1.618 (phi). This ratio is known as the Golden Ratio.

The architecture of Nautilus’ shell can be compared with a series of nested Golden Rectangles obtained when you snip off squares from a Golden Rectangle. If we connect the successive points where these whirling squares divide the sides in Golden Rations, we obtain a logarithmic spiral that coils inward toward the pole (the point given by the intersection of the diagonals, which was called fancifully “The eye of God”).

to continue press next